Scaled-space and image enhancement techniques based on parabolic Partial
Differential Equations (PDEs) have proved to be powerful methods in the
processing of two-dimensional (2D) and three-dimensional (3D) images and image
sequences. These models allow to include a-priori knowledge into the scale-space
evolution, and they lead to an image simplification which simultaneously
preserve or even enhance semantically important information such as edges,
lines, or flow-like structures. Refer to the
Mikula-Sarti-Sgallari papers for
the ideas behind the different approaches for image or image sequence analysis
as well as for the introduction of a new PDE model. Purpose of this research is
to analyze numerical schemes for these models for solving PDEs , based on
semi-implicit approximation in scale, finite-elements and finite volume in space
and to consider the numerical linear algebra aspects involved in the methods and
the related linear systems. Interesting applications of the PDE models for 2D
and 3D image analysis have been investigating such as automatic image sequence
restoration and image interpolation of medical images.
We have introduced new models for multiscale analysis of space-time image sequences (with application in echocardiography). The proposed nonlinear partial differential equations, representing the multiscale analysis, filters the sequence with keeping of the space-time coherent structures. They combine the ideas of regularized Perona-Malik anisotropic diffusion or geometrical diffusion of mean curvature flow type with Galilean invariant movie multiscale analysis of Alvarez, Guichard, Lions and Morel. The numerical method for solving the proposed partial differential equation was also suggested and its stability was shown.
Here,
we present some computational results with artificial and real image sequences.
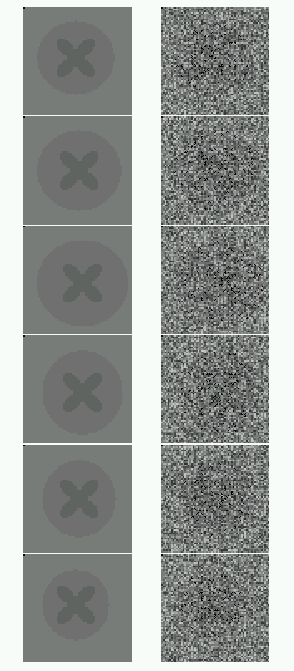
Figure 1. Original and noise-corupted
sequence of six 2D images.
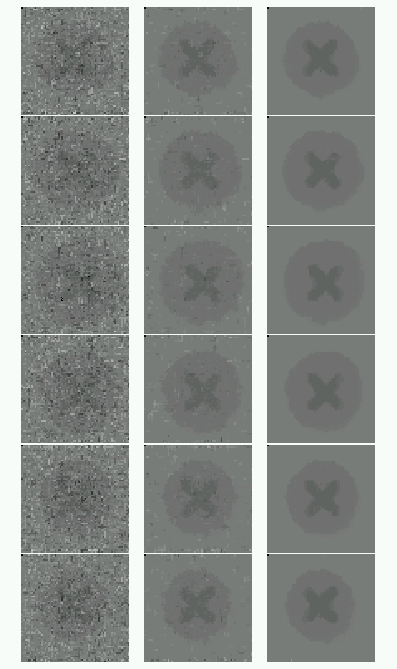
Figure 2. Reconstruction of the
sequence using our model. We plot 1st, 2nd and 5th scale steps in
columns.
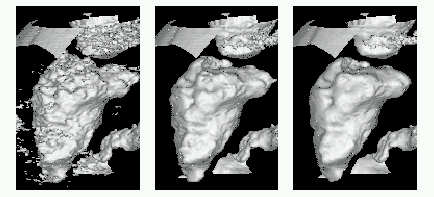
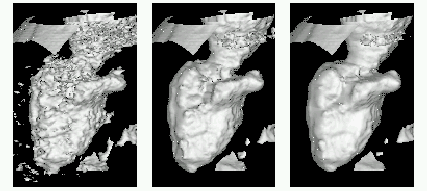
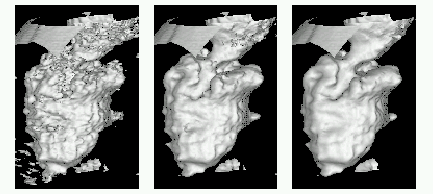
Figures 3a-c. The multiscale analysis of 1st, 5th and 9th time step of the echocardiographic sequence. The shape of the left
ventricle is extracted in those moments of cardiac cycle.
¨ A. SARTI, K.MIKULA, F. SGALLARI, C. LAMBERTI Nonlinear multiscale analysis models for filtering of 3D + time biomedical images, in "Geometric Methods in Bio-Medical image processing", R. Malladi, Ed., Lectures Notes Comput. Science and Eng., pp.107-127, Springer Verlag, 2002.
¨ A. SARTI, K.MIKULA, F. SGALLARI, C. LAMBERTI Evolutionary Partial Differential Equations for Medical Image Processing, Journal of Biomedical Informatics, pp. 77-91, Vol. 35, No. 2, April 2002.
¨ K. MIKULA, T. PREUSSER, M. RUMPF, F. SGALLARI On Anisotropic Diffusion in 3D image processing and image sequence analysis, in “Trends in Nonlinear Analysis”, (M.Kirkilionis, S. Kromker, R.Rannacher, F. Tomi (eds.), Springer Verlag, 2003, pp. 307-321.
